Non steady state solutions
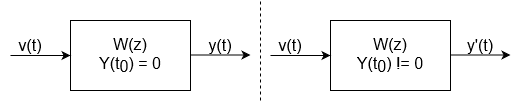
If we consider a stationary stochastic process $v(t)$ and an assintotically stable transfer function $W(z)$ then the steady state solution of the recursive equation defined by the transfer function $W(z)$ is a well defined stationary stochastic process $y(t)$.
If we now consider a differnt non-null initialization of the recursive equations the output $y'(t)$ is a different process, that is $y'(t)\ne y(t)$, and it is not stationary.
But since the transfer function is assintotically stable the different (not null) initialization tends to vanish over time: $$y'(t) \xrightarrow[t \to +\infty]{} y(t)$$ In particular the convergence to $y(t)$ is exponentially fast.